Source: Popular Mechanics

With all due respect to Pythagoras, newly uncovered evidence suggests that his famous theorem, used to calculate the lengths of each side of a right triangle, might be 1,000 years older than him. That, you know, sort of complicates the timeline.

For years, an Australian mathematician has been studying a 3,700-year-old tablet from the era of the ancient Babylonians. According to his analysis—published earlier this month in the journal Foundations of Science—the Babylonians have been using Pythagorean triples to divvy up and sell farmland far before the Greeks ever described that kind of math.

For those with misty, watercolored memories of their geometry and trigonometry lessons, Pythagoras is a star of trigonometry for his alleged discovery of the Pythagorean theorem, which states that “in a right-angled triangle the square of the hypotenuse is equal [to the sum of] the squares of the two other sides.”

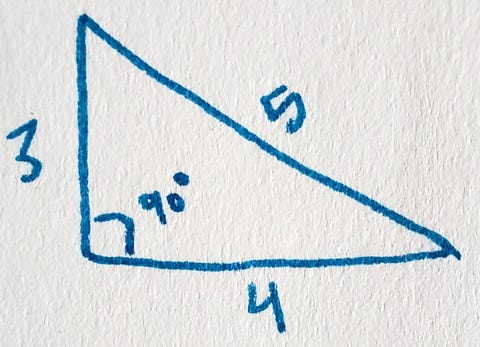
To solve for a hypotenuse (the longest side), you must start with a right triangle, which contains a 90-degree angle. From there, you square the length of each side. You should end up with a clean equation: the square of the hypotenuse c is equal to the sum of the squares of the other two legs a and b, so that a² + b² = c².

Contrary to what generations of pupils have learned in high school geometry, the Greek philosopher Pythagoras did not come up with this foundational theorem first. The proof lies in an ancient artifact that dates back to the Old Babylonian (OB) period: 1900 to 1600 B.C.

In 1894, Father Jean-Vincent Scheil excavated a clay tablet at an archaeological expedition at Sippar, southwest of Baghdad. “But its significance was not understood at the time,” Daniel Mansfield—lead author of the new research and a mathematician at the University of New South Wales in Sydney, Australia—writes in The Conversation.

The tablet, known as Si.427, is “the only known example of a cadastral document from the OB period, which is a plan used by surveyors to define land boundaries,” according to Mansfield. On the front of the artifact is an inscription showing a diagram of a field. The surveyors drew a series of perpendicular lines, using Pythagorean triples to create precise right angles so that the fields’ boundaries were as square as possible.

There’s evidence of triangles drawn around the periphery of the field documented on the tablet, but if that’s not enough proof, consider the back of the clay tablet. On it, cuneiform text describes the opposite side, including details about the sizes of the fields.

Mansfield notes that the key to Si.427, as well as another ancient tablet, named Plimpton 322, is the idea of regular numbers. Regular numbers are those whose prime factors only include 2, 3, and 5, making them very easy to multiply into larger, still compatible numbers. For a surveyor trying to find the closest length match, this is a key attribute.

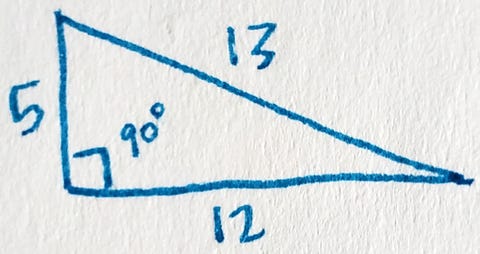
Surveyors used Pythagorean triples to measure fields and draw accurate maps, but some numbers that make up Pythagorean triples aren’t regular, and don’t make sense to try to scale up to fit any field. Plimpton 322 lists a bunch of Pythagorean triples and notes which of their three values is regular, helping ancient Babylonian surveyors to quickly “do the math,” so to speak.

What does all of this mean for our understanding of ancient peoples and their math?

It’s too late to rename Pythagorean triples to “Babylonian triples,” but now we know their use dates back to at least 1900 B.C. This displaces the previous earliest timeframe, which is the use of Pythagorean triples in ancient India dating back to about as early as 800 B.C. Both early usages are for extremely practical purposes, compared with the ancient Greeks’ use of Pythagorean triples to study the cosmos.

Pythagoras may still be still the father of geometry, but even he would admire the applied geometry being done to help build fire altars in India or split up fields in Babylon.

Source: Popular Mechanics